https://m.youtube.com/watch?v=8K-9FrSNVtc
Learn more about Math. Love math and Learn Math. Let's all learn together for another lesson about Gen Math
Monday, October 15, 2018
Deferred Annuity
What it is:
A deferred annuity is a type of annuity that delays monthly or lump-sum payments until an investor-specified date. The interest usually grows tax-deferred before it is withdrawn.
How it works (Example):
There are two phases in the life of a deferred annuity: the savings or accumulation phase, and the income or annuitization phase. During the accumulation phase, the investor will deposit money into the account either periodically or all in one lump-sum. When the annuity reaches the contractually agreed-upon date, the investor will begin receiving several payments over a period of time or in one lump-sum.
It is important to note that earnings on a deferred annuity are only taxed when they're withdrawn. However, if an investor withdraws money before the contractually agreed-upon date, he or she may have to pay considerable surrender fees. Annuity owners younger than 59.5 years old may also have to pay a 10% penalty tax, even if the surrender period has expired.
Another important feature of deferred annuities is the family protection, or death benefit, which guarantees that, should the owner die during the accumulation phase, the beneficiary will receive at least the amount of the owner's investments minus withdrawals, or the current market value of the account.
Often, the beneficiary cannot take advantage of a "step-up" in basis on an annuity, meaning that he or she becomes responsible for paying income taxes on all the gains in the account since it was opened.
It is important to note that earnings on a deferred annuity are only taxed when they're withdrawn. However, if an investor withdraws money before the contractually agreed-upon date, he or she may have to pay considerable surrender fees. Annuity owners younger than 59.5 years old may also have to pay a 10% penalty tax, even if the surrender period has expired.
Another important feature of deferred annuities is the family protection, or death benefit, which guarantees that, should the owner die during the accumulation phase, the beneficiary will receive at least the amount of the owner's investments minus withdrawals, or the current market value of the account.
Often, the beneficiary cannot take advantage of a "step-up" in basis on an annuity, meaning that he or she becomes responsible for paying income taxes on all the gains in the account since it was opened.
Why it Matters:m
Investors purchase deferred annuities for many reasons, the most common being the tax deferral of earnings, the lack of restrictions on the amount of the annual investment (unlike an IRA or 401(k)), and the guarantee of a lifelong annual income. Control over the investment decisions and the ability to switch between investments is also attractive to some investors.
Despite these advantages, wise investors must consider some important drawbacks to annuities. For one, annuity investors pay taxes on gains at their ordinary income taxrate (rather than the lower long-term capital gains tax rate) when they make withdrawals.
Fees are also major source of controversy for annuities. There are often front-end loads, state taxes, annual fees based on a percentage of the account value, early withdrawal penalties, etc., and they may offset much or all of an annuity's tax advantages. Variable annuities are particularly controversial because fees charged by their underlying mutual fundsalso burden them.
This is why less-than-stellar returns can turn into serious setbacks for some annuity investors. And even though investors can trade within their annuities and defer the taxes on the realized gains, most investors do not trade frequently enough to make this a huge advantage.
Pressuring sales tactics and less-than-transparent disclosure have also tarnished the image of annuities, so wise investors should be sure to read these disclosure materials and ask his or her financial consultant plenty of questions.
Ultimately, the appropriateness of an annuity is dependent on the investor's financial goals, tax situation, and the types of annuities available. Inflation and interest rate expectations may affect the type of annuity an investor chooses, as will the investor's wishes for his or her dependents
Despite these advantages, wise investors must consider some important drawbacks to annuities. For one, annuity investors pay taxes on gains at their ordinary income taxrate (rather than the lower long-term capital gains tax rate) when they make withdrawals.
Fees are also major source of controversy for annuities. There are often front-end loads, state taxes, annual fees based on a percentage of the account value, early withdrawal penalties, etc., and they may offset much or all of an annuity's tax advantages. Variable annuities are particularly controversial because fees charged by their underlying mutual fundsalso burden them.
This is why less-than-stellar returns can turn into serious setbacks for some annuity investors. And even though investors can trade within their annuities and defer the taxes on the realized gains, most investors do not trade frequently enough to make this a huge advantage.
Pressuring sales tactics and less-than-transparent disclosure have also tarnished the image of annuities, so wise investors should be sure to read these disclosure materials and ask his or her financial consultant plenty of questions.
Ultimately, the appropriateness of an annuity is dependent on the investor's financial goals, tax situation, and the types of annuities available. Inflation and interest rate expectations may affect the type of annuity an investor chooses, as will the investor's wishes for his or her dependents
Compound Interest
What is 'Compound Interest'
Compound interest (or compounding interest) is interest calculated on the initial principal and also on the accumulated interest of previous periods of a deposit or loan. Thought to have originated in 17th century Italy, compound interest can be thought of as “interest on interest,” and will make a sum grow at a faster rate than simple interest, which is calculated only on the principal amount. The rate at which compound interest accrues depends on the frequency of compounding such that the higher the number of compounding periods, the greater the compound interest. Thus, the amount of compound interest accrued on $100 compounded at 10% annually will be lower than that on $100 compounded at 5% semi-annually over the same time period.
Compounding as the Basis of Future Value
The formula for the future value (FV) of a current asset relies on the concept of compound interest. It takes into account the present value of an asset, the annual interest rate, and the frequency of compounding (or number of compounding periods) per year and the total number of years. The generalized formula for compound interest is:
FV = PV x [1 + (i / n)] (n x t), where:
- FV = future value
- PV = present value
- i = the annual interest rate
- n = the number of compounding periods per year
- t = the number of years
Example of Increased Compounding Periods
The effects of compounding strengthen as the frequency of compounding increases. Assume a one-year time period. The more compounding periods throughout this one year, the higher the future value of the investment, so naturally, two compounding periods per year are better than one, and four compounding periods per year are better than two.
To illustrate this effect, consider the following example given the above formula. Assume that an investment of $1 million earns 20% per year. The resulting future value, based on a varying number of compounding periods, is:
- Annual compounding (n = 1): FV = $1,000,000 x [1 + (20%/1)] (1 x 1) = $1,200,000
- Semi-annual compounding (n = 2): FV = $1,000,000 x [1 + (20%/2)] (2 x 1) = $1,210,000
- Quarterly compounding (n = 4): FV = $1,000,000 x [1 + (20%/4)] (4 x 1) = $1,215,506
- Monthly compounding (n = 12): FV = $1,000,000 x [1 + (20%/12)] (12 x 1) = $1,219,391
- Weekly compounding (n = 52): FV = $1,000,000 x [1 + (20%/52)] (52 x 1) = $1,220,934
- Daily compounding (n = 365): FV = $1,000,000 x [1 + (20%/365)] (365 x 1) = $1,221,336
As evident, the future value increases by a smaller margin even as the number of compounding periods per year increases significantly. The frequency of compounding over a set length of time has a limited effect on an investment's growth. This limit, based on calculus, is known as continuous compounding and can be calculated using the formula:
FV = PV x e (i x t), where e = the irrational number 2.7183.
In the above example, the future value with continuous compounding equals: FV = $1,000,000 x 2.7183 (0.2 x 1) = $1,221,40
Compound Interest Formula - Explained
Article Category: Finance | 320 Comments
Since I launched my compound interest calculator, I have regularly been the recipient of emails asking me to explain the formula for calculating compound interest. For that reason, we're going to tackle this subject today.
The concept of compound interest is that interest is added back to the principal sum so that interest is earned on that added interest during the next compounding period. If you would like more information on what compound interest is, please see the article what is compound interest?. For now, let's look at the formula and go through an example.
Annual compound interest formula
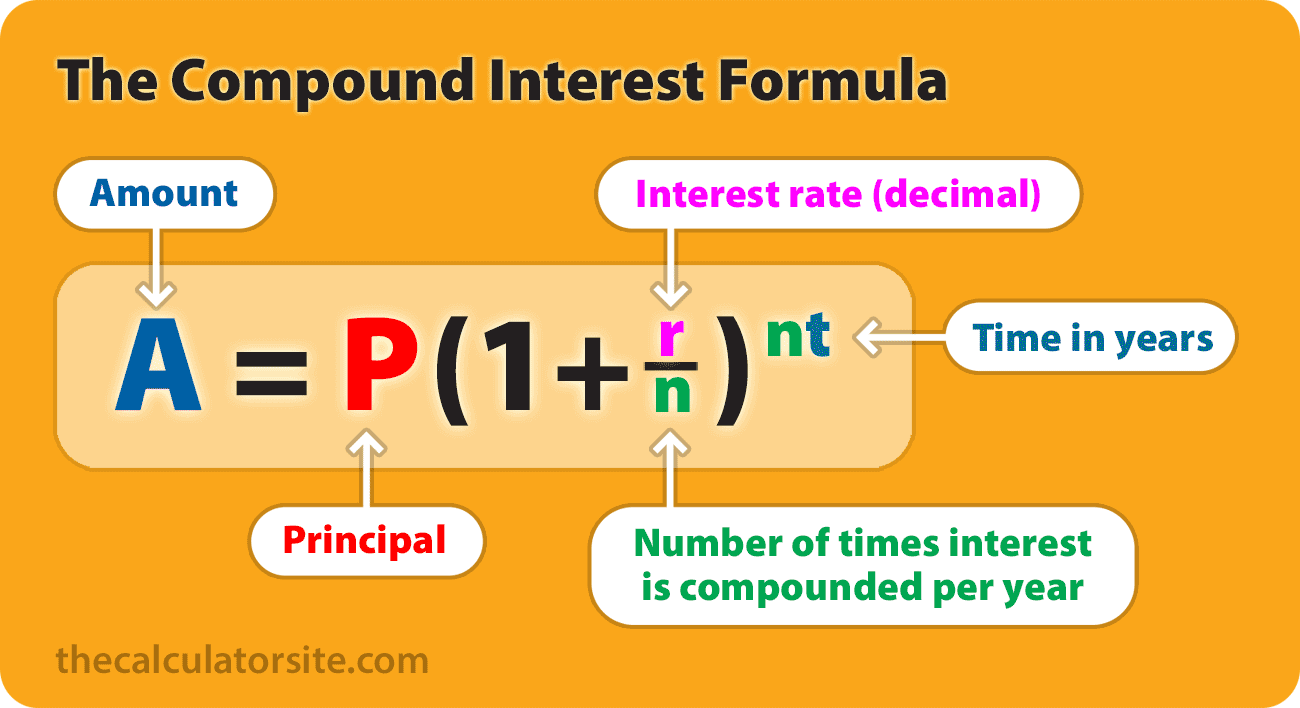
The formula for annual compound interest, including principal sum, is:
A = P (1 + r/n) (nt)
A = P (1 + r/n) (nt)
Where:
A = the future value of the investment/loan, including interest
P = the principal investment amount (the initial deposit or loan amount)
r = the annual interest rate (decimal)
n = the number of times that interest is compounded per year
t = the number of years the money is invested or borrowed for
P = the principal investment amount (the initial deposit or loan amount)
r = the annual interest rate (decimal)
n = the number of times that interest is compounded per year
t = the number of years the money is invested or borrowed for
Note that this formula gives you the future value of an investment or loan, which is compound interest plus the principal. Should you wish to calculate the compound interest only, you need this:
Total compounded interest = P (1 + r/n) (nt)- P
Let's look at an example
Compound interest formula (including principal):
A = P(1+r/n)(nt)
If an amount of $5,000 is deposited into a savingsaccount at an annual interest rate of 5%, compounded monthly, the value of the investment after 10 years can be calculated as follows...
P = 5000. r = 5/100 = 0.05 (decimal). n = 12. t = 10.
If we plug those figures into the formula, we get the following (note that ^ indicates 'to the power of'):
A = 5000 (1 + 0.05 / 12) ^ (12(10)) = 8235.05.
So, the investment balance after 10 years is $8,235.05.
Methodology
A few people have written to me asking me to explain step-by-step how we get the 8235.05. This all revolves around PEMDAS (also known as BODMAS in the UK) and the order of operations. Let's go through it:
A = 5000 (1 + 0.05 / 12) ^ (12(10))
(note that ^ means 'to the power of')
Using the order of operations we work out the totals in the brackets first. Within the first set of brackets, you need to do the division first and then the addition (division and multiplication should be carried out before addition and subtraction). We can also work out the 12(10). This gives us...
A = 5000 (1 + 0.00416) ^ 120
(note that the over-line in the calculation signifies a decimal that repeats to infinity. So, 0.00416666666...)
Then:
A = 5000 (1.00416) ^ 120
The exponent goes next. So, we calculate (1.00416) ^ 120.
This means we end up with:
5000 × 1.6470095042509848
= 8235.0475.
You may have seen some examples giving a formula of A = P ( 1+r ) ^ t . This simplified formula assumes that interest is compounded once per period, rather than multiple times per period.
The benefit of compound interest
The full benefit of compound interest will become clear when I tell you that without it, your investment balance in the above example would be only $7,500 ($250 per year for 10 years, plus the original $5000) by the end of the term.
So, thanks to the wonder of compound interest, you will gain an additional $735.05.
Interactive compound interest formula
Use the calculator below to show the formula and resulting compound interest calculation for your chosen figures. Note that this calculator requires JavaScript to be enabled in your browser.
P(1 +rn)(nt)=A
For a comprehensive set of tools for calculating compound interest on your savings, please see our compound interest calculators.
On the next page we look at the formula for compound interest with monthly contributions (how you can add a regular, additional monthly deposit) and the future value formula.
I
Compound interest for principal:
P(1+r/n)(nt)
Future value of a series:
PMT × {[(1 + r/n)(nt) - 1] / (r/n)}
also share information about your use of our site with advertising and analytics partners. Read our privacy policy.
Menu
You are here: the calculator site » articles » compound interest formula - explained
Compound Interest Formula - Explained
(continued from page 1...)
We continue this article with a look at how to add additional contributions to the compound interest formula.
Compound interest with regular additional contributions - formulae
A lot of people have asked me to include a single formula for compound interest with monthly additions. Believe me when I tell you that it isn't quite as simple as it sounds. In order to work out calculations involving monthly additions, you will need to use two formulae - our original one, listed on the first page of this article, plus the 'future value of a series' one for the monthly additions. At the request of readers, I've adapted the formula explanation to allow you to calculate periodic additions, not just monthly (added May 2016).
If the additional deposits are made at the END of the period (end of month, year, etc), here are the two formulae you will need:
Compound interest for principal:
P(1+r/n)(nt)
Future value of a series:
PMT × {[(1 + r/n)(nt) - 1] / (r/n)}
If the additional deposits are made at the BEGINNING of the period (beginning of year, etc), here are the two formulae you will need:
Compound interest for principal:
P(1+r/n)(nt)
Future value of a series:
PMT × {[(1 + r/n)(nt) - 1] / (r/n)} × (1+r/n)
Where:
A = the future value of the investment/loan, including interest
P = the principal investment amount (the initial deposit or loan amount)
PMT = the monthly payment
r = the annual interest rate (decimal)
n = the number of compounds per period (months, years, etc)
t = the number of periods (months, years, etc) the money is invested or borrowed
P = the principal investment amount (the initial deposit or loan amount)
PMT = the monthly payment
r = the annual interest rate (decimal)
n = the number of compounds per period (months, years, etc)
t = the number of periods (months, years, etc) the money is invested or borrowed
Let's put this into our example:
An amount of $5,000 is deposited into a savings account at an annual interest rate of 5%, compounded monthly, with additional deposits of $100 per month (made at the end of each month). The value of the investment after 10 years can be calculated as follows...
P = 5000. PMT = 100. r = 5/100 = 0.05 (decimal). n = 12. t = 10.
If we plug those figures into the formulae, we get:
Total = [ Compound interest for principal ] + [ Future value of a series ]
Total = [ P(1+r/n)^(nt) ] + [ PMT × (((1 + r/n)^(nt) - 1) / (r/n)) ]
Total = [ 5000 (1 + 0.05 / 12) ^ (12 × 10) ] + [ 100 × (((1 + 0.00416)^(12 × 10) - 1) / (0.00416)) ]
Total = [ 5000 (1.00416) ^ (120) ] + [ 100 × (1.00416)^(120) - 1) / 0.00416) ]
Total = [ 8235.05 ] + [ 100 × (0.647009497690848 / 0.00416) ]
Total = [ 8235.05 ] + [ 15528.23 ]
Total = [ $23,763.28 ]
Total = [ P(1+r/n)^(nt) ] + [ PMT × (((1 + r/n)^(nt) - 1) / (r/n)) ]
Total = [ 5000 (1 + 0.05 / 12) ^ (12 × 10) ] + [ 100 × (((1 + 0.00416)^(12 × 10) - 1) / (0.00416)) ]
Total = [ 5000 (1.00416) ^ (120) ] + [ 100 × (1.00416)^(120) - 1) / 0.00416) ]
Total = [ 8235.05 ] + [ 100 × (0.647009497690848 / 0.00416) ]
Total = [ 8235.05 ] + [ 15528.23 ]
Total = [ $23,763.28 ]
So, the investment balance after 10 years is $23,763.28.
You can learn more about this future value of a series formula, and use an interactive formula for it, in the future value formula article.
One thing you might notice is that this figure may differ slightly from the figure you get from the compound interest calculator. The reason for this is that the compound interest formula above assumes that the interest calculation occurs before the regular deposit is added on. The calculator, conversely, adds the deposit in first before calculating the interest. Both are legitimate ways of calculating.
For further examples of compound interest formulae for periodic compounding, monthly payments, mortgages and loans, I recommend taking a look at this Wikipedia article.
Other formulae
If you want to work backwards and find out how much you would need to start with in order to achieve a chosen future value, try the following version of the formula: P = A / ( 1 + r/n ) ^ (nt).
Let's say your goal is to end up with $10,000 in 5 years, and you can get an 8% interest rate on your savings, compounded monthly. Your calculation would be: P = 10000 / (1 + 0.08/12) ^ (12×5) = $6712.10. So, you would need to start off with $6712.10 to achieve your goal.
Scientific calculator
Need a scientific calculator to help with your calculations? Give this one from web2.0calc.com a try...
Compound interest with regular additional contributions - formulae
A lot of people have asked me to include a single formula for compound interest with monthly additions. Believe me when I tell you that it isn't quite as simple as it sounds. In order to work out calculations involving monthly additions, you will need to use two formulae - our original one, listed on the first page of this article, plus the 'future value of a series' one for the monthly additions. At the request of readers, I've adapted the formula explanation to allow you to calculate periodic additions, not just monthly (added May 2016).
If the additional deposits are made at the END of the period (end of month, year, etc), here are the two formulae you will need:
Compound interest for principal:
P(1+r/n)(nt)
Future value of a series:
PMT × {[(1 + r/n)(nt) - 1] / (r/n)}
If the additional deposits are made at the BEGINNING of the period (beginning of year, etc), here are the two formulae you will need:
Compound interest for principal:
P(1+r/n)(nt)
Future value of a series:
PMT × {[(1 + r/n)(nt) - 1] / (r/n)} × (1+r/n)
Where:
A = the future value of the investment/loan, including interest
P = the principal investment amount (the initial deposit or loan amount)
PMT = the monthly payment
r = the annual interest rate (decimal)
n = the number of compounds per period (months, years, etc)
t = the number of periods (months, years, etc) the money is invested or borrowed
Let's put this into our example:
An amount of $5,000 is deposited into a savings account at an annual interest rate of 5%, compounded monthly, with additional deposits of $100 per month (made at the end of each month). The value of the investment after 10 years can be calculated as follows...
P = 5000. PMT = 100. r = 5/100 = 0.05 (decimal). n = 12. t = 10.
If we plug those figures into the formulae, we get:
Total = [ Compound interest for principal ] + [ Future value of a series ]
Total = [ P(1+r/n)^(nt) ] + [ PMT × (((1 + r/n)^(nt) - 1) / (r/n)) ]
Total = [ 5000 (1 + 0.05 / 12) ^ (12 × 10) ] + [ 100 × (((1 + 0.00416)^(12 × 10) - 1) / (0.00416)) ]
Total = [ 5000 (1.00416) ^ (120) ] + [ 100 × (1.00416)^(120) - 1) / 0.00416) ]
Total = [ 8235.05 ] + [ 100 × (0.647009497690848 / 0.00416) ]
Total = [ 8235.05 ] + [ 15528.23 ]
Total = [ $23,763.28 ]
So, the investment balance after 10 years is $23,763.28.
You can learn more about this future value of a series formula, and use an interactive formula for it, in the future value formula article.
One thing you might notice is that this figure may differ slightly from the figure you get from the compound interest calculator. The reason for this is that the compound interest formula above assumes that the interest calculation occurs before the regular deposit is added on. The calculator, conversely, adds the deposit in first before calculating the interest. Both are legitimate ways of calculating.
For further examples of compound interest formulae for periodic compounding, monthly payments, mortgages and loans, I recommend taking a look at this Wikipedia article.
A lot of people have asked me to include a single formula for compound interest with monthly additions. Believe me when I tell you that it isn't quite as simple as it sounds. In order to work out calculations involving monthly additions, you will need to use two formulae - our original one, listed on the first page of this article, plus the 'future value of a series' one for the monthly additions. At the request of readers, I've adapted the formula explanation to allow you to calculate periodic additions, not just monthly (added May 2016).
If the additional deposits are made at the END of the period (end of month, year, etc), here are the two formulae you will need:
Compound interest for principal:
P(1+r/n)(nt)
Future value of a series:
PMT × {[(1 + r/n)(nt) - 1] / (r/n)}
If the additional deposits are made at the BEGINNING of the period (beginning of year, etc), here are the two formulae you will need:
Compound interest for principal:
P(1+r/n)(nt)
Future value of a series:
PMT × {[(1 + r/n)(nt) - 1] / (r/n)} × (1+r/n)
Where:
A = the future value of the investment/loan, including interest
P = the principal investment amount (the initial deposit or loan amount)
PMT = the monthly payment
r = the annual interest rate (decimal)
n = the number of compounds per period (months, years, etc)
t = the number of periods (months, years, etc) the money is invested or borrowed
P = the principal investment amount (the initial deposit or loan amount)
PMT = the monthly payment
r = the annual interest rate (decimal)
n = the number of compounds per period (months, years, etc)
t = the number of periods (months, years, etc) the money is invested or borrowed
Let's put this into our example:
An amount of $5,000 is deposited into a savings account at an annual interest rate of 5%, compounded monthly, with additional deposits of $100 per month (made at the end of each month). The value of the investment after 10 years can be calculated as follows...
P = 5000. PMT = 100. r = 5/100 = 0.05 (decimal). n = 12. t = 10.
If we plug those figures into the formulae, we get:
Total = [ Compound interest for principal ] + [ Future value of a series ]
Total = [ P(1+r/n)^(nt) ] + [ PMT × (((1 + r/n)^(nt) - 1) / (r/n)) ]
Total = [ 5000 (1 + 0.05 / 12) ^ (12 × 10) ] + [ 100 × (((1 + 0.00416)^(12 × 10) - 1) / (0.00416)) ]
Total = [ 5000 (1.00416) ^ (120) ] + [ 100 × (1.00416)^(120) - 1) / 0.00416) ]
Total = [ 8235.05 ] + [ 100 × (0.647009497690848 / 0.00416) ]
Total = [ 8235.05 ] + [ 15528.23 ]
Total = [ $23,763.28 ]
Total = [ P(1+r/n)^(nt) ] + [ PMT × (((1 + r/n)^(nt) - 1) / (r/n)) ]
Total = [ 5000 (1 + 0.05 / 12) ^ (12 × 10) ] + [ 100 × (((1 + 0.00416)^(12 × 10) - 1) / (0.00416)) ]
Total = [ 5000 (1.00416) ^ (120) ] + [ 100 × (1.00416)^(120) - 1) / 0.00416) ]
Total = [ 8235.05 ] + [ 100 × (0.647009497690848 / 0.00416) ]
Total = [ 8235.05 ] + [ 15528.23 ]
Total = [ $23,763.28 ]
So, the investment balance after 10 years is $23,763.28.
You can learn more about this future value of a series formula, and use an interactive formula for it, in the future value formula article.
One thing you might notice is that this figure may differ slightly from the figure you get from the compound interest calculator. The reason for this is that the compound interest formula above assumes that the interest calculation occurs before the regular deposit is added on. The calculator, conversely, adds the deposit in first before calculating the interest. Both are legitimate ways of calculating.
For further examples of compound interest formulae for periodic compounding, monthly payments, mortgages and loans, I recommend taking a look at this Wikipedia article.
Other formula
If you want to work backwards and find out how much you would need to start with in order to achieve a chosen future value, try the following version of the formula: P = A / ( 1 + r/n ) ^ (nt).
Let's say your goal is to end up with $10,000 in 5 years, and you can get an 8% interest rate on your savings, compounded monthly. Your calculation would be: P = 10000 / (1 + 0.08/12) ^ (12×5) = $6712.10. So, you would need to start off with $6712.10 to achieve
If you want to work backwards and find out how much you would need to start with in order to achieve a chosen future value, try the following version of the formula: P = A / ( 1 + r/n ) ^ (nt).
Let's say your goal is to end up with $10,000 in 5 years, and you can get an 8% interest rate on your savings, compounded monthly. Your calculation would be: P = 10000 / (1 + 0.08/12) ^ (12×5) = $6712.10. So, you would need to start off with $6712.10 to achieve
Y
Y
 report this ad
report this ad
Egg

